XYLENE POWER LTD.

SPHEROMAK STRUCTURE:

By Charles Rhodes, P.Eng., Ph.D.

SPHEROMAK CONCEPT:
The existence of stable quantum charged atomic and nuclear particles is enabled by a natural electro-magnetic structure known as a spheromak, in which electric and magnetic forces cancel each other at a quasi-toroidal surface known as a spheromak wall. Net charge circulating around a complex quasi-toroidal closed filament path on the spheromak wall surface forms the electric and magnetic field energy density distributions required for spheromak existence. The electric and magnetic fields of a spheromak maintain its stable geometry and contain a stable amount of energy.
 

SPHEROMAK EXISTENCE:
Spheromaks physically exist and have been photographed in plasmas. They anecdotally exist as ball lightning that has been ocassionally observed in the proximity of high altitude aircraft. They are believed to be the electro-magnetic structure that enables the existence of quantum charged particles such as electrons and protons.

Spheromaks can exist when due to a net charge and a circulating current within the spheromak wall, the toroidal magnetic field energy density at the inner surface of the spheromak wall equals the sum of the radial electric field energy density plus the poloidal magnetic field energy density at the outer surface of the spheromak wall. In these circumstances the average field energy density inside the spheromak wall is less than the field energy density that would pertain if the spheromak wall did not exist. Hence a spheromak is a potential energy well, which gives the spheromak structure long term stability.
 

SPHEROMAK COMPONENTS:
The essential components of a spheromak are: net charge that circulates around a multi-turn quasi-toroidal path at the speed of light, internal toroidal magnetic field energy, external poloidal magnetic field energy and external radial electric field energy. The circulating net charge forms a filament that has both toroidal and poloidal turns around the surface of the quasi-toroid. This surface is known as the spheromak wall. The field energy density at the inner surface of the spheromak wall exactly equals the field energy density at the outer surfce of the spheromak wall.
 

SPHEROMAK FIELDS:
For an isolated spheromak in a vacuum, at the exact center of the spheromak (at the origin) the net electric field is zero and the magnetic field is purely poloidal. In the region inside the spheromak wall the magnetic field is purely toroidal and the electric field is zero. In the region outside the spheromak wall the magnetic field is purely poloidal and the electric field is quasi-radial being locally normal to the spheromak wall. In the far field the electric field is spherically radial. The current circulates along a mixed toroidal and poloidal path defined by the charged filament winding that forms the spheromak wall.

The spheromak's field structure allows semi-stable plasma spheromaks and discrete stable atomic charged particles to exist and act as local stores of energy.
 

SPHEROMAK GEOMETRY:
A spheromak is cylindrically symmetric about the spheromak Z axis (major axis of symmetry) and is mirror symmetric about the spheromak's equatorial plane where Z = 0.

Photographs of a plasma spheromak are shown in the paper:
Spheromak formation and sustainment studies at the sustained physics experiment using high-speed imaging and magnetic diagnostics by Romero- Talamas et al , Physics of Plasmas, vol.13 (2006).

The theoretical cross sectional shape of a spheromak wall with Rs = 4 Rc is shown in Figure _____

LOCATION IN A SPHEROMAK:
A spheromak wall has both cylindrical symmetry about its main axis of symmetry and has mirror symmetry about its equatorial plane. Any position in a spheromak can be defined by:
(R, Z)
where:
R = radius from the main axis (Z axis) of cylindrical symmetry;
and
Z = height above (or below) the spheromak equatorial plane.
 

Conceptually a spheromak wall is a quasi-toroidal shaped closed surface, like the glaze on the surface of a doughnut, formed by the closed filament current path of a spheromak. This filament winding defines the spheromak wall surface curvature. In plan view, looking along the Z axis, a spheromak wall is round at both its inner and outer radii. In cross section, looking along the spheromak minor axis, the spheromak cross section is a distorted circle, thicker at the outside near Rw = Rs and thinner at the inside near Rw = Rc.
 

SPHEROMAK WALL EQUATION:
The general equation defining the position of a spheromak wall in cylindrical coordinates is:
1 / (Zw^2 + Rw^2)^2 = A / Rw^2 - B^2
where A and B are positive constants and the wall radius Rw meets the inequality:
Rw^2 < (A / B^2).

Nominal Spheromak Radius Ro is given by:
2 Ro^2 = Rs^2+ Rc^2

An important feature of a spheromak is that its shape remains constant independent of changes in its nominal radius Ro. Its stored energy is inversely proportional to Ro.

There are a number of important mathematical identities relating to spheromak wall geometry.

Then:
Rs^2 + Rc^2 = 2 Ro^2
FIX FROM HERE [Rs^2 - Rc^2] / Ro^2= A / 2 B ????[ Rs^2 Rc^2 = Ro^4
Rs^2 / Rc^2 =______ The spheromak size varies linearly with Ro.
The spheromak shape is controlled by the constant : (A / 2 B)
which typically about:
(Rs / Rc) = 4
 

SPHEROMAK HEIGHT Ho:

For the case of:
(Rs - Rc) = 4 Ro
H^2 = Ho^2 {(R / Ro) - [4 (R - Ro) /4 Ro]^2}

The corresponding X values where H = 0 are:
X = {-3 +/- [(9) - 4 (-1)(-1)]^0.5} / (- 2) = (1.5) +/- [9 - 4]^0.5 /2
=1.5 +/- 1.1180

Hence the range of validity is:
0.382 < X < 2.6180  

SPHEROMAK WALL:
Outside the spheromak wall the field energy density U has electric and poloidal magnetic field components.
Thus outside the spheromak wall:
U = Up + Ue
= ([Bp(R, Z)]^2 / 2 Muo) + ([Epsilono / 2] [E(R, Z)]^2)

Inside the spheromak wall the field is purely toroidal magnetic:
U = Ut(R)
= (Muo / 2) [Nt I / 2 Pi Ro]^2 [Ro / R]^2

At the spheromak wall:
Ut(R) = Up(R, Z) + Ue(R, Z)
or
([Bp(R, Z)]^2 / 2 Muo) + ([Epsilono / 2] [E(R, Z)]^2) = (Muo / 2) [Nt I / 2 Pi Ro]^2 [Ro / R]^2

This equation defines the position of the spheromak wall. The functions [Bp(R, Z)]^2 and [Ep(R, Z)]^2 are developed in terms of Ro on the web page titled Theoretical spheromak.

At R = Rc, Z = 0:
Utc = Btc^2 / 2 Muo
= (Bpc^2 / 2 Muo) + (Epsilono / 2) Eec^2]
and at R = Rs, Z = 0
Uts = Bts^2 / 2 Muo
= (Bps^2 / 2 Muo) + (Epsilono / 2) Ees^2]

Bto = Muo Nt I / 2 Pi Ro
or
Uto = Muo Nt^2 I^2 / 8 Pi^2 Ro^2
and
Utc = [Muo Nt^2 I^2 / 8 Pi^2 Ro^2] [Ro^2 / Rc^2]
and
Uts = [Muo Nt^2 I^2 / 8 Pi^2 Ro^2] [Ro^2 / Rs^2]
 

SPHEROMALK WINDING:
Let Np = number of poloidal winding turns,
Let Nt = number of toroidal winding turns
Let Lp = length of a single purely poloidal winding turn,
Let Lt = length of a single purely toroidal wining turn.
Let Lh = length of closed winding
Then:
Lh^2 = Lp^2 + Lt^2 [(Np Lp) / (Nt Lt)] = Mp / Mt
where Mp an Mt are integers and where
P = Mp + 2 Mt, where P = prime number
and
{(Np Lp) / (Nt Lt)] ~ (1 / 2) for real spheromaks.

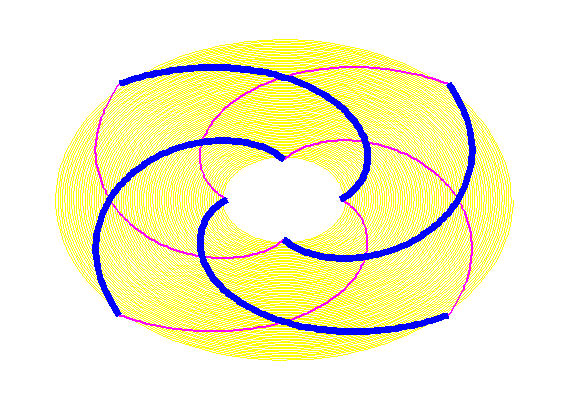
SPHEROMAK WINDING CONCEPT:
An approximate plan view of the current path (filament) of a theoretical elementary spheromak with Np = 3 and Nt = 4 is shown below. The blue lines show the current path on the upper face of the spheromak. The red lines show current path on the lower face of the spheromak. Note that the current path never intersects itself except at the point where the current starts to retrace its previous path.

In the diagram yellow shows the region of toroidal magnetic field. Outside the yellow region is a poloidal magnetic field and a spherically radial electric field.

This elementary spheromak winding pattern was generated using a polar graph and formulae of the form:
R = Rc + K [t - t(2N)] where t(2N) = (2 N) (3 Pi / 4) and t(2N) < t < t(2N+1)
and
R = Rs - K [t - t(2N + 1)]
where:
t(2N + 1) = (2N + 1)(3 Pi / 4)
and
t(2N + 1) < t < t(2N + 2)
where:
N = 0, 1, 2, 3. Use:
Rc = 1000,
Rs = 4105,
K = (4140 / Pi)
Top to bottom connection points were depicted by adjusting the torus Rs to 4045 and Rc to 1060.
 

ATOMIC PARTICLE SPHEROMAKS:
Atomic particle spheromaks have a quantized charge that superficially appears to be at rest with respect to an inertial observer. Isolated stable atomic particles such as electrons and protons hold specific amounts of energy (rest mass). When these particles aggregate with opposite charged particles the assembly emits photons. This photon emission decreases the total amount of energy in the assembly, creating a mutual potential energy well.

In an atomic particle spheromak net charge moves at the speed of light C around a closed spiral filament path of length Lh. The spheromak net charge Qs is uniformly distributed along this current path. The uniform charge distribution along the current path and the uniform current cause constant electric and magnetic fields. The time until an element of net charge retraces its previous path is (1 / F) where:
F = C / Lh
is the characteristic frequency of the spheromak. Note that frequency F increases as filament length Lh decreases.

An isolated spheromak in free space has a distorted circular cross section. However, the fields of an atomic particle spheromak may be further distorted by externally imposed electric and magnetic fields.
 

SPHEROMAK CROSS SECTIONAL DIAGRAM:
The following diagram shows the approximate cross sectional shape of a spheromak.

For this diagram: Ho = 2
and
(Rs - Rc)= 3 Ro

Note that the cross section of a real spheromak is distorted elliptical, not round.

In this diagram on the main axis of symmetry is R = 0

At R = 0, Z = 0 the field energy density is maximum and is entirely due to the poloidal magnetic field.  

GEOMETRICAL FEATURES OF A SPHEROMAK:
Important geometrical features of a spheromak include:
Rc = the spheromak wall inside radius on the equatorial plane;
Rs = the spheromak wall outside radius on the equatorial plane;
Ro = the radius of an imaginary ring radius on the equatorial plane which indicates the nominal linear size of a spheromak;
Ho = the spheromak wall height above the equatorial plane at R = Ro;
Np = number of poloidal filament turns about the major axis of symmetry;
Nt = number of toroidal filament turns about the minor axis in the spheromak;

The subscript c refers to spheromak wall inside radius (core) on the equatorial plane;
s refers to the spheromak wall outside radius on the equatorial plane.

When the spheromak filament has passed through the spheromak central hole Nt times it has also circled around the main axis of spheromak symmetry Np times, and has reached the point in its closed path where it originally started.

Define:
Lt = one purely toroidal filament turn length;
Lp = 2 Pi Ro = one purely poloidal filament turn length;
 

SPHEROMAK FILAMENT PATH LENGTH Lh:
Electromagnetic spheromaks arise from the electric current formed by distributed net charge Qs circulating at the speed of light C around the closed spiral path of length Lh which defines the spheromak wall. On the equatorial plane the spheromak inner wall minimum radius is Rc and the spheromak's outer wall maximum radius is Rs.

Let Np be the integer number of poloidal turns in filament length Lh and let Nt be the integer number of toroidal turns in filament length Lh.

The spheromak wall contains Nt toroidal turns equally spaced around 2 Pi radians about the main spheromak axis of symmetry.
Each purely quasi-toroidal winding turn has length:
Lt
so the purely quasi-toroidal spheromak winding length is:
(Nt Lt)

The spheromak wall contains Np poloidal turns which are spaced around the spheromak wall perimeter. The spheromak field calculations are based on the poloidal turns being concentrated at R = Ro. The purely poloidal turn length is:
Lp = 2 Pi Ro
and the purely poloidal winding length is:
(Np Lp)

The total spheromak winding length Lh is:
Lh^2 = (Np Lp)^2 + (Nt Lt)^2

In one spheromak cycle period T the poloidal angle advances Np (2 Pi) radians. In the same spheromak cycle period T the toroidal angle advances Nt (2 Pi) radians.
In each case the frequency remains constant. Hence:
(poloidal angle advance) / (toroidal angle advance) = d(Phi) / d(Theta) = [Np / Nt] average

Note that to prevent spheromak collapse, Np and Nt cannot be equal and cannot have common integer factors.
 

SPHEROMAK SHAPE PARAMETERS:
The relative shape of a spheromak is defined by the ratios:
(Rc / Ro), (Rs / Ro) and (Ho / Ro)

The winding of a spheromak is defined by the number of poloidal filament turns Np and the number of toroidal filament turns Nt.

The energy and frequency of a spheromak are a function of Lh. The ratio (Lh / Ro) is the same for all isolated spheromaks.
 

SPHEROMAK PARAMETER DEFINITIONS:
Define:
R = radial distance of a point from the major axis of symmetry of spheromak;
Z = axial distance of a point above the spheromak equatorial plane (Z is negative for points below the equatorial plane);
H = distance of a point on the spheromak wall above the spheromak equatorial plane;
Ro = characteristic spheromak radius
Ho = H|(R = Ro)
Hm = H|(R = Rm)
Ue = electric field energy density as a function of position outside the spheromak wall;
Up = magnetic field energy density as a function of position outside the spheromak wall;
U = Ue + Up + Ut = total field energy density at any position;
Upor = (Bpo^2 / 2 Muo) = magnetic field energy density at R = 0, Z = 0;
Lp = 2 Pi Ro
Lt = 2 Pi K Ro = single turn toroidal winding length
Utc = toroidal field energy density at R = Rc, Z = 0
Ut = Uto (Ro / R)^2
= toroidal magnetic energy density function inside the spheromak wall
Uts = Uto (Ro / Rs)^2 = toroidal magnetic energy density at R = Rs
Utc = Uto (Ro / Rc)^2 = toroidal magnetic energy density at R = Rc
Upor = _________
= poloidal magnetic field energy density at the origin on the spheromak axis.
 

SPHEROMAK FILAMENT PARAMETERS
Define:
Ih = filament current;
Lh = overall length of closed filament loop;
Dh = center to center distance between adjacent filament paths
As = outside surface area of spheromak wall
Q = proton net charge
Qs = net charge on spheromak
Nnh = integer number of negative charge quanta
Nph = integer number of positive charge quanta
Vn = velocity of negative charge quanta along charge hose
Vp = velocity of positive charge quanta along charge hose
C = speed of light
Nr = Np / Nt
= ratio of two integers which have no common factors. This ratio must be inherently stable.
 

SPHEROMAK CHARGE DISTRIBUTION ASSUMPTION:
Assume that the spheromak charge is uniformly distributed over the filament length Lh.
 

EQUATORIAL PLANE:
On the spheromak's equatorial plane:
Z = 0
For points on the spheromak's equatorial plane the following statements can be made:

For R = 0 the net electric field is zero;
For R < Rc the toroidal magnetic field is zero;
For R < Rc the magnetic field Bp is purely poloidal;
For R = 0 the magnetic field is parallel to the axis of cylindrical symmetry;

For Rc < R < Rs the electric field is zero;
For Rc < R < Rs the poloidal magnetic field is zero;
For Rc < R < Rs the toroidal magnetic field Bt is proportional to (1 / R).

For Rs < R in free space the electric field Ero is spherically radial;
For Rs < R in the far field the electric field Ero is proportional to (1 / R^2);
For Rs < R in free space the toroidal magnetic field is zero;
For Rs < R in the far field the poloidal magnetic field Bp is proportional to (1 / R^3);
 

FILAMENT CURRENT:
Ih = [Qp Np Vp + (- Q Ne Ve)] / Lh
= Q C / Lh
 

SPHEROMAK FILAMENT WINDING GEOMETRY:
A very important issue in understanding natural spheromaks is grasping that:
Np cannot equal Nt and that Np and Nt can have no common factors other than one. Otherwise the windings would fall on top of one another or the spheromak would collapse.
 

SPHEROMAK FILAMENT PATH:
Consider a quasi-toroidal winding with a poloidal axial length at radius Ro of 2 Pi Ro.

This winding has Np round poloidal turns effectively located at radius Ro at Z = 0 and has Nt quasi-toroidal turns centered at radius:
Rx = [(Rs + Rc) / 2].
These toroidal turns have a horizontal radius of:
[(Rs - Rc) / 2].

Hence the wall to wall width of the spheromak at its equator is:
(Rs - Rc)
and its height is:
2 Ho.

The minor axis of the spheromak toroidal winding lies along R = Rx, Z = 0 .

Rx = [(Rs + Rc)/ 2]

Any point on this winding can be identified by its Theta and Phi values. Theta is the angle about the spheromak minor axis Rx measured with respect to a radial line from Rx to the orgin. Phi is the angle about the major axis of symmetry measured with respect to the same radial line. Hence at the filament closure point:
Phi = 0, Theta = 0, or Phi = 2 Pi Np, Theta = 2 Pi Nt,
where Np and Nt are positive integers with no common integer factors.

The range of Phi is:
0 < Phi < 2 Pi Npo radians;
The range of Theta is:
0 < Theta < 2 Pi Nto radians

The average value of dTheta / dPhi is given by:
dTheta / dPhi = Nto / Npo

In a spheromak dTheta / dPhi is nearly constant at its operating point.

A very important issue is that dL / d(Theta) is always positive. Hence if Theta advances with time so also does L. The net charge moves along the filament at the constant speed of light C.

The filament winding path is partly described by:
R = Rx + [(Rs - Rc) / 2] cos(Theta)
where
Rx = (Rs + Rc) / 2
and
H = Ho sin(Theta)

dY = R d(Phi)

dL^2 = (dH)^2 + (dR)^2 + (dY)^2

R = Rx + [(Rs - Rc) / 2] cos(Theta)
or
R^2 = Rx^2 + 2 Rx [(Rs - Rc) / 2] cos(Theta) + [(Rs - Rc) / 2]^2 cos^2(Theta)

Hence:
R^2 (Np^2 / Nt^2)
= Rx^2 (Np^2 / Nt^2) + Rx [(Rs - Rc)] cos(Theta)(Np / Nt)^2 + [Np^2 / Nt^2][(Rs - Rc) / 2]^2 cos^2(Theta)

Differentiation of R gives:
dR = - [(Rs - Rc) / 2] sin(Theta) d(Theta)
 

However:
[Lh]^2 = Nt^2 [Lt]^2 + Np^2 {Lp}^2

This expression is carried forward to the web page titled: Spheromak Winding Constraints and to the web page titled: Theoretical Spheromak.
 

This web page last updated on August 23, 2024.

Home	Energy Physics	Nuclear Power	Electricity	Climate Change	Lighting Control	Contacts	Links